When observed from the orbital path of the Earth, the sun appears to move in relation to the fixed stars, following the ecliptic as its annual path on the celestial sphere. This cycle repeats itself, lasting slightly longer than 365 days.
The ecliptic represents the plane of the Earth’s orbit around the Sun. [1] [2] [a] From the vantage point of an observer on Earth, the Sun’s movement across the celestial sphere throughout the year aligns with the ecliptic against the backdrop of the stars. [3] The ecliptic serves as a significant reference plane and forms the foundation of the ecliptic coordinate system.
- 1 The Sun’s visible motion
- 2 Relationship to the celestial equator
- 3 Tilt of the ecliptic
- 4 The plane of the solar system
- 5 The celestial reference plane
- 6 Eclipses
- 7 Equinoxes and solstices
- 8 In constellations
- 9 Astrology
- 10 See also
- 11 Notes and References
- 12 external link
Visible motion of the Sun
Due to the Earth’s orbit around the Sun, as well as the Moon’s orbit around the Earth, the apparent path of the Sun appears to fluctuate slightly over the course of about one month. Additionally, the Earth-Moon barycenter is affected by the gravitational pull of another planet in the solar system, causing it to wobble around its center position in a complex manner. This wobbling motion results in the ecliptic, which is the Sun’s apparent path throughout the year. [4]
Because it takes the Earth approximately one year to complete its orbit around the Sun, the Sun’s apparent position takes one year to complete a full revolution around the ecliptic. Due to the Earth’s orbit, the Sun moves just under 1° to the east each day, resulting in a small difference in its position relative to the stars. This causes any given spot on the Earth’s surface to align directly north or south of the Sun about four minutes later each day compared to a hypothetical Earth that does not orbit. As a result, a day on Earth lasts 24 hours instead of the approximately 23 hours and 56 minutes of a sidereal day. It is important to note that this is a simplified explanation based on a constant-speed orbit of the Earth around the Sun. In reality, the Earth’s orbital velocity varies slightly throughout the year, leading to variations in the apparent speed at which the Sun moves along the ecliptic. For instance, the Sun is located north of the celestial equator for approximately 185 days per year and south of it for about 180 days. These changes in orbital velocity contribute to the equation of time.
Connection to the celestial equator
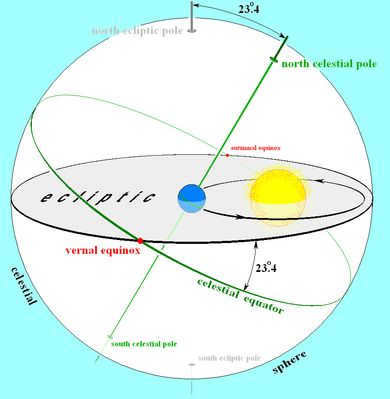
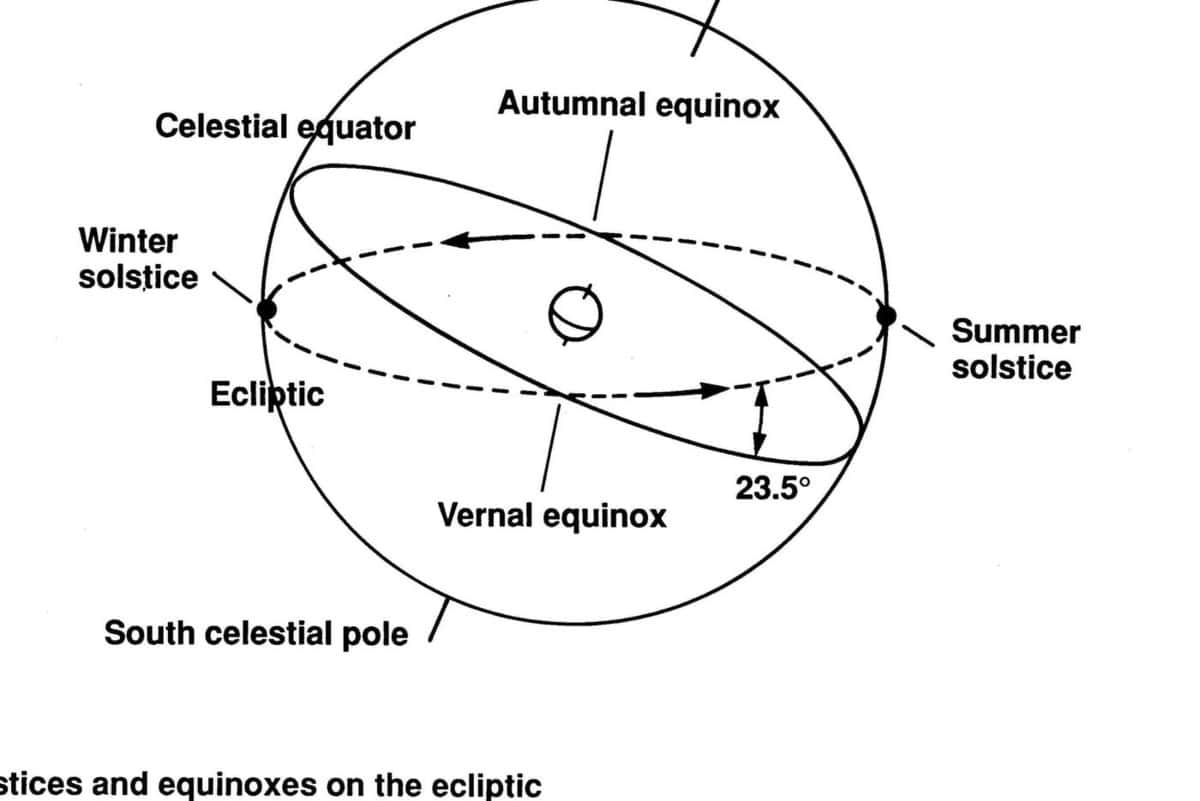
The reference plane known as the ecliptic is formed by the intersection of the Earth’s orbit projected in all directions with the celestial sphere. In the image above, the ecliptic plane is shown projected outward (gray) onto the celestial sphere, along with Earth’s equator and polar axis (green). This plane intersects the celestial sphere along a great circle (black), which is the same circle that the Sun appears to orbit the Earth. The points where the ecliptic and equator intersect on the celestial sphere are the spring and fall equinoxes (red), marking the times when the Sun appears to cross the celestial equator.
Due to the fact that the Earth’s axis of rotation is not perpendicular to its orbital plane, the equatorial plane of the Earth is not on the same plane as the plane of the ecliptic. Instead, it is tilted at an angle of approximately 23.4°, which is referred to as the ecliptic tilt. [8] When the equator is projected onto the celestial sphere, creating the celestial equator, it intersects with the ecliptic at two points known as the equinoxes. As the sun appears to move along the ecliptic, it crosses the celestial equator at these points, one from south to north and the other from north to south. [5] The south-to-north crossing is called the vernal equinox, also known as the first point of Aries and the ascending node of the ecliptic on the celestial equator. [9] The north-to-south crossing is known as the autumnal equinox or descending node.
Orientation Luni-solar precession is the process by which the earth’s axis and equator, which are not fixed in space, rotate around the pole of the ecliptic every 26,000 years. This phenomenon is primarily caused by the gravitational influence of the moon and sun on the Earth’s equatorial bulge. The ecliptic itself is also not fixed, as it experiences a small movement due to gravitational perturbations from other celestial bodies in the solar system, known as planetary precession. When these two motions combine, they create what is known as General precession, which results in a shift of the equinoxes by approximately 50 angular seconds (or 0.014 degrees) per year. [10]
Once again, this is a simplification. The periodic movements of the Moon and the apparent periodic movements of the Sun (which are actually caused by the Earth’s orbit) create short-term periodic oscillations of the Earth’s axis and, consequently, the celestial equator. These oscillations are known as nutation. [11] Nutation introduces a periodic element to the positions of the equinoxes. The positions of the celestial equator and the (vernal) equinox, taking into account both precession and nutation, are referred to as the true equator and equinox. On the other hand, the positions without nutation are called the mean equator and equinox. [12]
The tilt of the ecliptic refers to the angle at which the Earth’s equator is tilted in relation to the ecliptic, or the Earth’s axis of rotation in relation to the perpendicular of the ecliptic. Currently, the tilt is approximately 23.4° and is gradually decreasing by 0.013 degrees (47 angular seconds) per century due to the influence of planetary perturbations. [13]
Astronomers determine the precise value of the tilt by observing the movements of the Earth and other planets over an extended period of time. As observations become more accurate and our understanding of celestial dynamics improves, astronomers generate updated ephemerides that provide various astronomical values, including the tilt.
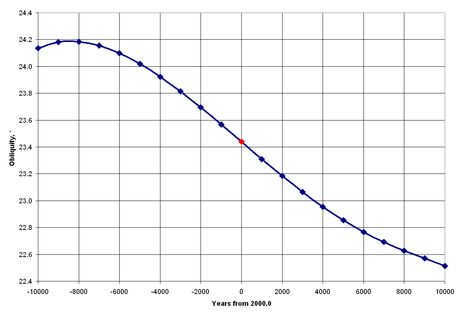
The tilt of the ecliptic changes over a span of 20,000 years, as shown in the diagram from Laskar (1986) [14]. It is interesting to note that during this time, the tilt only varies between 24.2° and 22.5°. The red dot on the diagram represents the year 2000.
Prior to 1983, the slope for any given date was calculated using the research of Newcomb, who studied the positions of the planets until around 1895:
ε = 23° 27′ 08″.26 – 46″.845 Т – 0″.0059 Т 2 + 0″.00181 Т 3
In the above equation, ε represents the slope and Т represents the tropical ages from B1900.0 to the specific date in question [15].
Since 1984, the Jet Propulsion Laboratory’s DE series of computer ephemerides have taken over as the primary ephemerides from the Astronomical Almanac. The inclination based on DE200, which analyzed observations from 1911 to 1979, was calculated as follows:
ε = 23° 26′ 21″.45 − 46″.815 Т − 0″.0006 Т 2 + 0″.00181 Т 3
where the value of Т represents the Julian ages from J2000.0. [16]
JPL’s basic ephemerides are continuously updated. The Astronomical Almanac for 2010 indicates: [17]
ε = 23° 26′ 21″.406 − 46″.836769 Т − 0″.0001831 Т 2 + 0″.00200340 Т 3 − 0″.576×10−6 Т 4 − 4″.34×10−8 Т 5
These equations for the slope angle are highly accurate for a relatively short period of time, possibly several centuries. [18] J. Laskar derived an equation to order Т 10 which is accurate to 0″.04 per 1000 years over 10,000 years. [14]
All of these phrases are designed to bear in mind the inclination, i.e., the tilting of the equator without any wobbling. In the true or instantaneous inclination, nutation is also taken into account. [19]
The Plane of the Solar System
 | ||
| The planets Mercury, Venus, Earth, and Mars are all aligned along the ecliptic plane, which is the same plane in which the Earth revolves around the sun. This alignment can be seen from both top and side views. | In July 2010, four planets were lined up along the ecliptic, providing a visual demonstration of how they revolve around the sun in a similar plane. This photo was taken at sunset in Surakarta, Java, Indonesia. | |
The majority of the main celestial bodies in the solar system revolve around the sun in a nearly identical plane. This is likely connected to the way in which the solar system was formed from a protoplanetary disk. The current representation of this disk, known as the the unchanging plane of the solar system, is perhaps the closest approximation we have. The orbit of Earth, and consequently the path of its orbit known as the ecliptic, is slightly tilted in relation to the unchanging plane by just over 1 degree. Jupiter’s orbit is also slightly off, deviating by a mere half a degree, while the other major planets deviate by approximately 6 degrees. As a result, most celestial bodies in the solar system appear to be positioned very near to the ecliptic when observed from Earth.
The unchanging plane is determined by the total angular momentum of the whole solar system, which is essentially the combined vector sum of all the orbital and rotational angular momenta of all the celestial bodies in the system; more than 60% of this total is attributed to the orbit of Jupiter. [20] Calculating this sum requires precise knowledge of every object in the system, making it a somewhat uncertain value. Due to the uncertainty surrounding the exact location of the fixed plane and the fact that the ecliptic is clearly defined by the apparent movement of the sun, the ecliptic is used as the reference plane for the solar system for the sake of accuracy and convenience. The only drawback to using the ecliptic as opposed to a fixed plane is that over geological time scales, it will shift in relation to fixed reference points in the distant celestial background. [21] [22]
Celestial reference plane
The motion of the sun along the ecliptic (red) can be seen on the inside of the celestial sphere. Ecliptic coordinates (red) are shown, while the celestial equator (blue) and equatorial coordinates (blue) appear to oscillate as the Sun moves forward, as they are tilted towards the ecliptic.
One of the major reference planes for positions on the celestial sphere is the ecliptic, along with the celestial equator. The ecliptic poles, perpendicular to the ecliptic, include the north pole of the ecliptic, which is located north of the equator. Compared to the celestial equator, the ecliptic is more stationary against the stars in the background, with its motion primarily due to planetary precession, accounting for approximately 1/100th of the motion of the celestial equator. [23]
Ecliptic coordinates are a convenient way to describe the locations of objects in the Solar System. This is because most planetary orbits have a slight tilt with respect to the ecliptic, meaning they always appear close to it in the sky. The Earth’s orbit, and thus the ecliptic, undergoes only minor movement, making it a relatively stable reference point in relation to the stars.
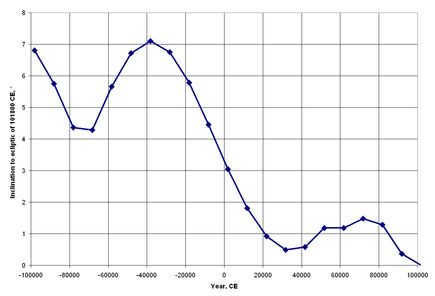
In Dzobek’s study from 1892, it was observed that the tilt of the ecliptic changes over a span of 200,000 years. [26] Specifically, in 101,800 A.D., the slope to the ecliptic was noted. Interestingly, during this period, the ecliptic only rotates about 7 degrees, while the celestial equator completes several full cycles around the ecliptic. It is worth mentioning that the ecliptic serves as a relatively stable reference point when compared to the celestial equator.
Due to the precessional movement of the equinoxes, the celestial coordinates of celestial objects are constantly changing. In order to specify a position in celestial coordinates, a specific equinox, known as the epoch, is used. The coordinates are relative to the direction of the equinox on that particular date. For instance, the Astronomical Almanac [27] provides the heliocentric position of Mars at 0h Earth time on January 4, 2010 as follows: longitude 118° 09' 15" 0.8, latitude + 1° 43' 16" 0.7, and true heliocentric distance of 1.6302454 astronomical units. These coordinates are based on the mean equinox and ecliptic date of January 4, 2010 0h TT, without taking nutation into account.
Eclipses
Due to the slight tilt of the Moon’s orbit (approximately 5.145°) in relation to the ecliptic, and the Sun’s proximity to the ecliptic, eclipses consistently take place either at or near it. It should be noted that eclipses do not occur during every conjunction and opposition of the Sun and Moon, but rather when the Moon is in close proximity to the ascending or descending node while also being in conjunction (new moon) or opposition (full moon). The ecliptic derived its name from the observation made by ancient civilizations that eclipses only occur when the Moon crosses it. [28]
Equinoxes and Solstices
The moments of the equinoxes and solstices are specific times when the sun’s apparent ecliptic longitude, including the effects of aberration and nutation, is at 0°, 90°, 180°, and 270°. Due to perturbations from Earth’s orbit and calendar anomalies, the dates of these events are not constant. [29]
An equirectangular chart displays the direct ascension of modern constellations, taking into account their declination. The graph includes a dotted line indicating the ecliptic, which is color-coded according to family and the year the constellations were established. This detailed view provides an in-depth look at the constellations and their relationship to the ecliptic.
Currently, the constellations that the ecliptic passes through are:
Astrology
The zodiac is a celestial belt about 20° wide, known as the ecliptic, through which the sun, moon, and planets appear to move. [31] This region is traditionally divided into 12 signs, each spanning 30° of longitude, which roughly corresponds to the sun’s movement in one month. [32] In ancient times, these signs aligned with the 12 constellations located on the ecliptic. [33] Although not always used in modern terminology, these signs still hold significance. “The first point of Aries” was originally named when the March equinox sun was in the constellation Aries, but due to the precession of the equinoxes, it has since moved to Pisces. [34]
Additional Resources
Important Information and Sources
- ^USNO Marine Almanac Office; UK Hydrographic Office, HM Marine Almanac Office (2008). Astronomical Almanac 2010. GPO. p. M5. ISBN978-0-7077-4082-9 .
- ^"LEVEL 5 Vocabulary and Glossary of Terms."
- ^"Ecliptic: the annual path of the Sun on the celestial sphere".
- ^ U.S. Naval Observatory Naval Almanac Office (1992). P. Kenneth Seidelmann (ed.). Explanatory appendix to the astronomical almanac. University Science Books, Mill Valley, California. ISBN0-935702-68-7 .
- , p. 11
- abc Directions north and south refer to the positions on the celestial sphere where the north celestial pole and south celestial pole are located. East is the direction in which the Earth rotates, while West is the opposite direction.
- ^Astronomical Almanac 2010, sec. C
- ^Explanatory appendix (1992), sec. 1,233
- ^Explanatory appendix (1992), pp. 733
- ^Astronomical Almanac 2010, items M2 and M6
- ^Explanatory Appendix (1992), secs. 1.322 and 3.21
- ^ U.S. Naval Observatory Naval Almanac Office; H.M. Naval Almanac Office (1961). Explanatory appendix to astronomical ephemerides and American ephemerides and the naval almanac. H.M. Stationery Office, London.
- , sec. 2C
- ^Explanatory appendix (1992), pages 731 and 737
- ^ Chauvenet, William (1906). A manual of spherical and practical astronomy. vol. J.B. Lippincott Co. of Philadelphia.
- , Fine Art. 365-367, pages 694-695, at Google Books
- ^ ab Laskar, J. (1986). “Secular terms of classical planetary theories utilizing results of the general theory of relativity”. Bibcode:1986A & A . 157 . 59L. Journal citation required journal = (help)
- , table 8, in SAO / NASA ADS.
- ^Explanatory Appendix (1961), section 2B
- ^ U.S. Naval Observatory, Office of Naval Almanac; H.M. Office of Naval Almanac (1989). Astronomical Almanac 1990. U.S. Government Printing Office. ISBN0-11-886934-5 .
- , page B18
- ^Astronomical Almanac 2010, p. B52
- ^ Newcomb, Simon (1906). A compendium of spherical astronomy. MacMillan Co., New York.
- , p. 226-227, in Google Books
- ^ Mius, Jean (1991). Astronomical algorithms. Willmann-Bell, Inc, Richmond, Virginia. ISBN0-943396-35-2 .
- , Ch. 21
- ^"The midplane (invariant plane) of the solar system passing through the barycenter". April 3, 2009 Archived from the original on June 3, 2013 . Retrieved April 10, 2009 .
- Produced with Vitagliano, Aldo. "Solex 10". Archived from the original (computer program) on April 29, 2009 . Retrieved April 10, 2009 .
- ^ Danby, J. M. A. (1988). Fundamentals of celestial mechanics. Willmann-Bell, Inc. in Richmond, Virginia. section 9.1. ISBN0-943396-20-4 .
- ^ Roy, A.E.. (1988). Orbital motion (3rd ed.). Publishers Institute of Physics. section 5.3. ISBN0-85274-229-0 .
- ^ Montenbrück, Oliver (1989). Practical calculations of ephemerides. Springer-Verlag. ISBN0-387-50704-3 .
- , sec 1.4.
- ^Explanatory appendix (1961), sec. 2А
- ^Explanatory appendix (1961), sec. 1G
- ^ Dziobek, Otto (1892). Mathematical theories of the motion of the planets. Register Publishing Co. , Ann Arbor, Mich.
- , p. 294, at Google Books
- ^Astronomical Almanac 2010, item E14.
- ^ Ball, Robert S. (1908). A treatise on spherical astronomy. Cambridge University Press. p.83.
- ^ Meeus (1991), ch. 26
- ^ Serviss, Garrett P. (1908). Astronomy with the naked eye. Harper & Brothers, New York and London. pp.105, 106.
- ^ Bryant, Walter W. (1907). A history of astronomy. p. 3. ISBN9781440057922 .
- ^ Bryant (1907), pp. 4.
- ^ See, for instance, Leo, Alan (1899). Astrology for all. L.N. Fowler and company. p.8. astrology.
- ^ Vallado, David A. (2001). Fundamentals of astrodynamics and applications (2nd ed.). El Segundo, CA: Microcosm Press. pp. 153. ISBN1-881883-12-4 .
External link
- Ecliptic: the annual path of the Sun on the celestial sphere Department of Physics, Durham University
- Seasons and ecliptic simulator University of Nebraska-Lincoln
- PICTURING THE SKY A brief guide to the celestial sphere James B. Kahler, University of Illinois
- Seasons of the Earth U.S. Naval Observatory
- The basics – ecliptic, equator, and coordinate systems AstrologyClub.Org
- Kinoshita, H .; Aoki, S. (1983). “Definition of the ecliptic”. Celestial Mechanics. 31 (4): 329-338. Bibcode:1983CeMec..31..329K. doi:10.1007 / BF01230290. S2CID122913096.
- A comparison of the definitions of Leverrier, Newcome, and Standish.
- Find out more about the ecliptic and its significance in astronomy at the Department of Physics, Durham University.
- Explore a seasons and ecliptic simulator at the University of Nebraska-Lincoln’s website.
- Learn about the celestial sphere and how to picture the sky with this brief guide by James B. Kahler from the University of Illinois.
- Discover the seasons of the Earth and their connection to the ecliptic with the U.S. Naval Observatory.
- Get an overview of the basics of the ecliptic, equator, and coordinate systems at AstrologyClub.Org.
- Read the article “Definition of the ecliptic” by H. Kinoshita and S. Aoki in the journal Celestial Mechanics.
- Compare the definitions of the ecliptic by Leverrier, Newcome, and Standish.
The examination of the characteristics of interplanetary space distant from the ecliptic plane holds significant scientific intrigue. The act of straying from the ecliptic plane necessitates additional energetic expenditure. These expenses fluctuate dramatically depending on which particular region outside the ecliptic plane we intend to investigate.
The most straightforward approach to infiltrating regions distant from the ecliptic plane is to do so at the periphery of the solar system. To accomplish this, it suffices to position an artificial planet in an outer elliptical orbit that is inclined at a minor angle to the ecliptic plane. Even a slight inclination would relocate the spacecraft to far-reaching distances from the Sun, spanning tens of millions of kilometers away from the ecliptic plane.
Penetrating space “above” and “below” the Sun presents a much greater challenge. Let’s consider the scenario where we attempt to launch an artificial planet into a circular orbit that is perpendicular to the ecliptic plane. In such an orbit, the artificial planet would intersect with the Earth six months after launch.
Take a look at Figure 134, which displays artificial planets in circular orbits with a radius of 1 astronomical unit (a. e.) at different inclinations.
For the artificial planet to achieve heliocentric escape velocity, it must have the same magnitude as the velocity of the Earth. By examining the construction in Figure 134, we can deduce that the geocentric escape velocity must also be equal to the initial escape velocity. In fact, we have determined that the initial escape velocity is even greater than the fourth space velocity.
An elliptical orbit can be achieved by a flight path that is perpendicular to the ecliptic plane, with the perihelion positioned just behind the Sun’s surface. To maintain this orbit, the spacecraft would need an initial velocity slightly higher than the fourth space velocity. However, the maximum distance of the spacecraft from the ecliptic plane, which is halfway between Earth and the Sun, would only be 0.068 astronomical units or approximately 10 million kilometers. This distance is considered too small in the context of the vastness of the Solar System, and the necessary launch speed is extremely difficult to attain!
However, it is relatively straightforward to explore regions situated many millions of kilometers “above” and “below” the Earth’s orbit. To place a man-made celestial body into a circular orbit with a radius of 1 astronomical unit (a.u.), whose plane is inclined at an angle to the ecliptic plane, we require a geocentric escape velocity. We can determine the angle from which we find the departure velocity from the Earth to be relatively small, yet sufficient to allow the artificial planet to move away from the Earth to a maximum distance of 26 million kilometers within 3 months of its launch (Fig. 134, b). It is worth noting that such an artificial planet, while moving alongside the Earth (albeit outside its sphere of influence), will still experience noticeable perturbations caused by our planet.
Start with an initial speed that matches the third cosmic velocity (which enables you to place the spacecraft in a circular orbit with a radius of 1 a. inclined at an angle of 24 ° to the ecliptic plane. The spacecraft’s maximum distance from Earth (in 3 months) will be 60 million.
A compilation of intriguing assignments and inquiries
А.
When it comes to the pole, the Sun spends half of the year above the horizon and the other half below it. But what about the Moon?
Б.
In order to address this query, it is crucial to first grasp the reasons behind the Sun’s continuous presence in the sky for half the year at the pole and how it behaves.
Earth and Moon’s Orbits
The Moon’s path and the Earth’s path are approximately in the same plane, known as the ecliptic plane. This plane is tilted at a specific angle in relation to the celestial equator, resulting in half of the ecliptic being situated above the equator (in the northern hemisphere of the sky) and the other half below the equator. At the pole, the celestial equator aligns with the horizon. As the Sun follows the ecliptic, completing a full rotation around the Earth in one year, it spends half of the year above the equator (and pole’s horizon) and the other half below the equator.
The Moon completes a full orbit around the Earth in approximately one month, following a similar path in nearly the same plane. Therefore, it remains visible in the polar sky for half of the month, and then disappears below the horizon for the remaining half.
On the day of the vernal equinox (with a slight variation of three days due to atmospheric refraction), the Sun makes its entrance into the sky at the pole. As the Earth rotates daily, the Sun creates circular patterns above the horizon. Simultaneously, as it moves along the ecliptic, the Sun gradually rises higher in the sky until reaching its peak at the summer solstice. This movement results in an upward spiral in the sky over a span of three months, equivalent to approximately ninety rotations. Subsequently, the Sun follows a similar descending spiral, ultimately disappearing below the horizon on the day of the fall equinox (or, more precisely, three days thereafter).
The Clementine lunar exploration spacecraft captured this image in 1994, clearly revealing the ecliptic plane. In the photo, the Moon is illuminated by Earth on the right side, while the Sun’s glare rises over the dark part of the Moon’s surface. Additionally, the lower left corner features three dots representing the planets Saturn, Mars, and Mercury.
The term “ecliptic” is derived from the Greek word “(linea) ecliptica” meaning “eclipse”. It refers to a large circle on the celestial sphere that marks the path of the visible annual motion. The “ecliptic plane” specifically pertains to the plane of the Earth’s orbit around the Sun. To be more precise, the modern definition of the ecliptic is the intersection of the celestial sphere with the plane of the Earth’s barycenter orbit.
Description
Because of the inclination of the Moon’s orbit in relation to the ecliptic and the Earth’s rotation around the barycenter of the Moon-Earth system, as well as the influence of other planets on the Earth’s orbit, the position of the true Sun does not always align precisely with the ecliptic, but can vary by a few seconds of arc. It can be said that the ecliptic represents the path followed by the “average Sun”.
While the tilt of the celestial equator plane changes relatively quickly, the ecliptic plane is more stable in relation to distant stars and quasars, although it does experience small changes due to perturbations from the planets of the solar system.
The term “ecliptic” is used because solar and lunar eclipses only occur when the Moon is near the points where its orbit intersects with the ecliptic. These points on the celestial sphere are known as lunar nodes, and their circulation along the ecliptic, which takes about 18 years, is called the saros or draconic period.
The ecliptic plane is the primary plane in the ecliptic celestial coordinate system.
The inclinations of the orbits of the planets in the solar system with respect to the ecliptic plane
The image captured by the Clementine lunar exploration spacecraft in 1994 vividly displays the presence of the ecliptic plane. In the photograph, the Moon is illuminated by the Earth on the right, while the Sun’s glare emerges over the Moon’s dark surface. Additionally, the planets Saturn, Mars, and Mercury are depicted as three dots in the lower left corner.
The term “ecliptic” is used to describe the phenomenon, which has been observed since ancient times, that solar and lunar eclipses occur when the Moon is close to the points where its orbit intersects with the ecliptic. These points are known as lunar nodes on the celestial sphere. The path of the ecliptic crosses through the zodiacal constellations and the Serpentine. The ecliptic plane is the main plane in the ecliptic celestial coordinate system.
Additional Resources
Wikimedia Foundation. 2010.
Explore other definitions of “Ecliptic plane” in various dictionaries:
The Laplace plane refers to a plane that passes through the center of mass of the solar system and is perpendicular to the momentum vector of the planetary motion. In other words, it is perpendicular to the vector that represents the total orbital momentum of all the planets and the rotational momentum… … Source: Wikipedia
The celestial sphere is divided by the celestial equator. It is an imaginary auxiliary sphere with an arbitrary radius onto which the celestial bodies are projected. This sphere is used to solve various astrometric problems. The center of the celestial sphere is… … Source: Wikipedia
A fundamental plane is a plane that determines various systems of coordinates, such as spherical, geographic, geodesic, and astronomical coordinates. It also determines the origin of coordinates at a specific point on this plane. This concept is mentioned in Wikipedia.
The Laplace’s normal plane is a plane that passes through the center of mass of the solar system and is perpendicular to the angular momentum vector. It was introduced by P. Laplace in 1789, who highlighted its advantages as the main coordinate system. This information can be found in the Big Soviet Encyclopedia.
The Deep Ecliptic Survey (DES) is a project that aims to search for Kuiper Belt objects. It utilizes the facilities of the National Optical Astronomy Observatory (NOAO) at Kitt Peak National Observatory. The project is led by Bob Millis and has been operational since… This project is mentioned in Wikipedia.
The image obtained in 1994 by the Clementine lunar exploration spacecraft clearly shows the plane of the ecliptic. In this image, the Moon is illuminated by the Earth on the right side, while the Sun’s glare rises above the dark background on the left side. This photograph was captured by Clementine’s camera and provides a unique perspective of the celestial bodies. (source: Wikipedia)
The celestial equator divides the celestial sphere into two hemispheres. This sphere is an imaginary auxiliary sphere with an arbitrary radius onto which the celestial luminaries are projected. It is used to solve various astrometric problems and serves as a reference point for the center of the celestial sphere. (source: Wikipedia)
The celestial equator marks the division of the celestial sphere. This sphere, which has an arbitrary radius, is an imaginary auxiliary sphere onto which the celestial luminaries are projected. It is a useful tool for solving astrometric problems and serves as a reference point for the center of the celestial sphere. (source: Wikipedia)
In articles about space and astronomy, the term “ecliptic” is frequently encountered. This term is used by scientists and astrologers to refer to the position of objects in space relative to the solar system and the orbits of celestial bodies within the system. So, what exactly is the ecliptic?
What is the connection between the zodiac and the ecliptic?
Ancient priests, while observing the celestial luminaries, noticed a peculiar behavior of the Sun. It seemed to be moving in relation to the stars. By tracking its movement across the sky, observers discovered that exactly one year later, the Sun always returned to its starting point. Moreover, the “route” it followed remained the same from year to year. This path is known as the ecliptic, which is the line along which our main luminary traverses the sky throughout the calendar year.
The stellar regions that the shining Helios, in his golden chariot drawn by golden horses (as imagined by the ancient Greeks), passes through.
The circle of 12 constellations that the Sun moves through was named the zodiac, and these constellations are referred to as zodiacal constellations.
If you happen to be a Leo, don’t bother looking up at the sky in July, the month of your birth. During this time, the Sun is located in your constellation, so unless you’re fortunate enough to witness a total solar eclipse, you won’t be able to see it.
The path of the Sun
If we observe the celestial sphere during daylight hours (which can be done not only during a total solar eclipse but also with a regular telescope), we can notice that the Sun occupies a specific position within one of the zodiacal constellations. For instance, in November, it’s likely to be found in the constellation Scorpio, while in August, it will be in Leo. The following day, the Sun’s position will slightly shift to the left, and this pattern repeats every day. Then, after a month (on November 22), the Sun will ultimately reach the boundary of the Scorpio constellation and transition into Sagittarius.
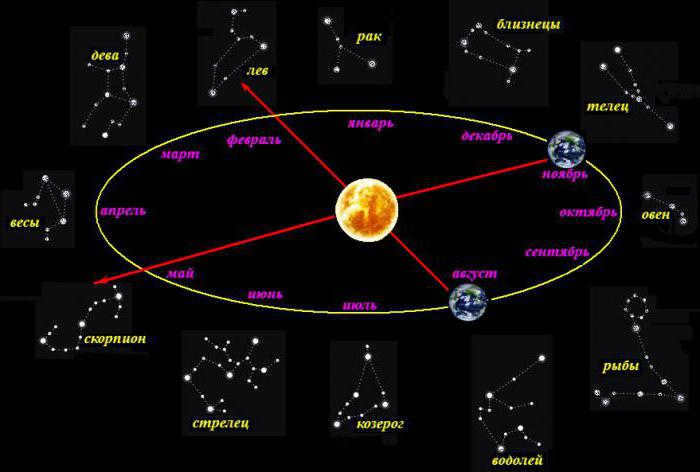
In August, it is evident from the diagram that the Sun will be within the boundaries of the Leo constellation. And so forth. By plotting the Sun’s position on the celestial chart daily, we will eventually have a map with a complete ellipse traced on it after a year. This is why this line is referred to as the ecliptic.
When to Make an Observation
However, to make an observation of the constellations under which a person is born, one should look in the month that is opposite to their date of birth. This is because the ecliptic, which is the path of the Sun, determines the visibility of the constellations. For example, if a person is born in August under the sign of Leo, the Leo constellation will be high above the horizon at noon, when the sunlight will obstruct its visibility.
Reasons for Using the Term “Ecliptic Plane”
Aside from its role in defining the Sun’s annual path, the ecliptic is commonly referred to as a plane. The term “ecliptic plane” is frequently employed to explain the spatial orientation of celestial bodies and their orbits. Let’s delve into its significance.
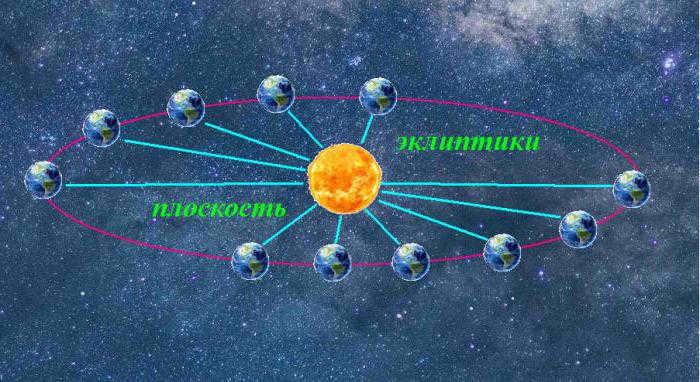
When we consider the orbit of our planet around its parent star and the lines that can be drawn from Earth to the Sun at different moments in time, we find that they all lie on a single plane known as the ecliptic. This plane can be thought of as an imaginary disk, with the 12 constellations described earlier located on its sides. If we draw a perpendicular line from the center of this disk, it will intersect with a point on the celestial sphere in the northern hemisphere. This point is located near both Ursa Major and Ursa Minor, two constellations commonly known as the Big and Little Dipper.
As we know, the Earth’s rotation axis is inclined to the ecliptic axis by 23.44 degrees. This inclination is responsible for the changing seasons on our planet.
And our “neighbors”
Here is a brief overview of the concept of the ecliptic. In the field of astronomy, scientists are also interested in studying the movements of other celestial bodies within our solar system. According to calculations and observations, it has been determined that all the major planets orbit the Sun in nearly the same plane.
The planet closest to the Sun, Mercury, stands out the most in this regard, with its plane of rotation deviating from the ecliptic by as much as 7°.
Among the outer planets, Saturn has the highest inclination angle for its orbit (approximately 2.5°). However, considering its vast distance from the Sun – ten times farther than Earth – this can be forgiven for such a massive celestial body.
On the other hand, the orbits of smaller celestial bodies such as asteroids, dwarf planets, and comets deviate much more significantly from the ecliptic plane. For example, Erid, the twin of Pluto, has an extremely elongated orbit.

When it approaches the Sun at its closest distance, this object gets even closer to the Sun than Pluto does, at a distance of 39 astronomical units (AU) – an astronomical unit is equal to the distance from the Earth to the Sun, which is approximately 150 million kilometers. After that, it moves back towards the Kuiper belt, reaching a maximum distance of almost 100 AU. As a result, its plane of rotation is inclined to the ecliptic by about 45 degrees.
We know that celestial bodies move in the sky, but their movement is not random; instead, it is confined to a narrow zone that divides space in half. This zone passes through the 12 constellations of the zodiac.
Below is a table showing the constellations that the Sun’s ecliptic crosses.
| Aries | April 19 – May 13 |
| Taurus | May 14 – June 19 |
| Gemini | June 20 – July 20 |
| Cancer | July 21 – August 9 |
| Leo | August 10 – September 15 |
| Virgo | September 16 – October 30 |
| Libra | October 31 – November 22 |
| Scorpio | November 23 – November 29 |
| Serpentine | November 30 – December 17 |
| Sagittarius | December 18 – January 19 |
| Capricorn | January 20 – February 15 |
| Aquarius | February 16 – March 11 |
| Pisces | March 12 – April 18 |
You can also determine the position of the Sun in the zodiac, just like ancient astronomers did. To accomplish this, you must observe which constellations cross the ecliptic of the celestial luminary, completing a full circle each year, as well as which of the final constellations of the zodiac rises before or after the Sun. Therefore, each monthly period throughout the year has its own zodiac sign.
Astrologers believe that the stars inexplicably influence life based on the sign under which a person is born. However, it is important to understand that the sign assigned to each month in the horoscope does not correspond to the constellation where the Sun is located during that month, but rather where it was situated in ancient times. This discrepancy is a result of the precession of the equinoxes.
Definitions of the sidereal zodiac
When it comes to defining the sidereal zodiac, different sidereal schools have different approaches. Here are the main ones:
Cyril Fagan’s zodiac
The Western Sidereal zodiac was established by Cyril Fagan and Donald Bradley in the late 1940s. They incorporated both archaeological and statistical methods in their research. Fagan initially proposed that Spica at 30° Virgo could be used as a reference star from the Indian system.
In Fagan’s zodiac, four major stars are used as reference points: in addition to Spica, which is known from the Indian system, three other stars or star groups are taken into consideration: Aldebaran, Antares, and Regulus. These are the original stars on which the zodiac is based. – Cyril Fagan
In 1956, Bradley utilized statistical analysis incorporating lunar data to calculate the precise offset of 0° 06′. By 1950, Spica had shifted to 29° 06′. The Synetic Point of the vernal equinox, also known as the stellar vernal equinox, was situated at 5° 07′28″ Pisces. According to Cyril Fagan, in 221 AD, the 0° sideric Aries aligned with the 0° tropical Aries. The Fagan-Bradley sideric zodiac for 1950 corresponds to an ayanamsa of 24° 02′, resulting in a deviation of 0° 53′ from the Indian zodiac. The western Fagan/Bradley zodiac presented here stems from our own research, drawing upon archaeological and statistical evidence.
The representation of the first group should include the Indian zodiac. Indian astrology, known as Jyotic, borrowed the foundational elements of astrology from the Babylonian-Egyptian culture and is based on the sideric zodiac. A point of reference is set at 30° Virgo or at the 0° scale with Spica, the ear. Presently, there are various schools within Indian astrology, each with slight variations in the definition of ayanamsa. The most commonly accepted ayanamsa dates as of January 1, 1950 are as follows:
Two prominent Indian teachers share an almost identical ayanamsa, while newer Indian astrologers deviate significantly from it.
Zodiac with unique sizes of constellations
The second method, known as the real zodiac, operates with varying lengths of individual constellations along the ecliptic, such as the constellation Virgo with an estimated ecliptic length of 44° or Libra with 23°. The IAU definitions are derived from this approach. The “Anthroposophical” Zodiac also adheres to this perspective and follows a phenomenological path.
3.3 Ecliptic coordinate system
When studying the motion of celestial bodies in the solar system, it is more convenient to use the ecliptic coordinate system instead of the equatorial coordinate system. This is because the orbital planes of most celestial bodies in the solar system are inclined at small angles to the plane of the Earth’s orbit (with Pluto’s orbit being the largest among the planets). Therefore, it is natural for an observer on Earth to choose the plane of the Earth’s orbit as the main plane of reference in the coordinate system.
The equations of dynamics, which describe the Earth’s movement around the Sun in its orbit, serve as the foundation for the construction of the ecliptic coordinate system. The ecliptic plane, which is the midplane of the Earth’s orbit, defines the path along which the Earth travels. This plane intersects with the celestial sphere, forming what is known as the ecliptic. As the Earth moves in its orbit, the Sun appears to move across the sky in relation to the distant stars. The ecliptic completes a full rotation in one year, causing the Sun to move relative to the stars at a rate of X degrees per day.
The Earth’s axis of rotation is tilted at an angle to the orbital plane. This angle also applies to the relationship between the equator and the ecliptic plane, which we’ll call the “ecliptic to equator” angle. This angle slowly changes due to the gravitational pull from other planets, causing perturbations in the Earth’s motion. As a result, the Earth’s center of mass appears to shift above and below the midplane of its orbit. Similarly, the center of the Sun also appears to be above and below the ecliptic as a reflection of these perturbations.
In the ecliptic coordinate system, the principal plane is known as the ecliptic plane. The north pole of the ecliptic is denoted by , and by definition, the arc must be smaller (Fig. ).
Definition 3.3.1.
The circle of latitude is defined as the great circle that passes through the poles of the ecliptic and a celestial object.
ecliptic latitude
The second coordinate is ecliptic
longitude, which represents the dihedral angle between the great circle passing through the poles of the ecliptic and the dynamic point of the spring equinox, and the circle of latitude:
The fundamental circles in the ecliptic coordinate system include the ecliptic, the circle of latitude, and the main points being the poles of the ecliptic and the point of the vernal equinox.
3.2 Equatorial Coordinate System
Table of contents
3.4. Galactic coordinate system >>
All publications on the same topic >>
Astrometry is the measurement of the positions and motions of celestial objects. It is an important part of astronomy and is used to study the structure and dynamics of the universe.
Astronomical instruments are the tools that astronomers use to observe and measure celestial objects. They include telescopes, cameras, spectrographs, and other specialized equipment.
Astronomical education is the study of astronomy and its related fields. It includes learning about the history of astronomy, the principles of astrophysics, and the techniques used in space exploration.
Astrophysics is the branch of astronomy that deals with the physics of celestial objects, including stars, galaxies, and the universe as a whole. It combines principles from physics and astronomy to understand the nature and behavior of these objects.
History of astronomy is the study of the development of astronomy throughout human history. It includes the discoveries and contributions of ancient civilizations, as well as the advancements made during the scientific revolution and the modern era.
Astronautics and space exploration are fields that focus on the exploration and study of space. They involve the design and operation of spacecraft, as well as the study of celestial bodies and phenomena.
Amateur astronomy is a hobby that involves observing and studying celestial objects with the use of amateur telescopes and other equipment. It is a popular pastime for many people interested in astronomy.
Planets and the Solar System are the study of the planets and other objects that orbit the Sun. It includes the study of their physical characteristics, orbits, and interactions with each other.
The Sun is the star at the center of our Solar System. It is a source of light, heat, and energy, and plays a crucial role in the dynamics of the Solar System.
Individual evidence
- John David North: A History of Astronomy and Cosmology Vieweg, 1 background
- John M. Steele: Observation and prediction of the timing of eclipses by early astronomers, Dordrecht / Boston / London, 2000, pp. 176
- Alfred Fork: The World Concept of the Chinese, New York, 1975, p. 1998.
- John David North: A History of Astronomy and Cosmology Vieweg, 2 Ancient Egypt, pp. 8-11
- B.L. van der Waarden: Science for Adults, pp. 78
- B.L. van der Varden: Science for adults, pp. 124
- B.L. van der Varden: Science for adults, pp. 258-261.
- Translated by Carl Manitius, book one, chapter sixteen
- George Thibault: Astronomy, astrology, and mathematics in the field of Indo-Aryan philology and ancient times, Strasbourg, 1899, page 12
- George Thibault: Astronomy, Astrology, and Mathematics, page 13
- Daniel Martin Varisco: Medieval Agriculture and Islamic Science, Seattle/London 1994
- John David North: A History of Astronomy and Cosmology Vieweg, 8 Der Ostliche Islam., pages 122-126
- John David North: A History of Astronomy and Cosmology Vieweg, 8 Der Ostliche Islam, pages 126-128
- Hans Gunther Zekl: Nikolaus Kopernikus: The New Worldview, Hamburg 1990, page XLVIII.
- Ernst Zinner: Origin and Propagation of the Copernican Doctrine, Munich 1988, Peuerbach, Regiomontan
- Nicolaus Copernicus: De Revolutionibus, 1543, 2nd book, 2nd chapter or 3rd book, 10th chapter.
- Andreas Gutmann: Introduction to celestial mechanics and the calculation of ephemerides. 1994, p. 160.
- Axel D. Wittmann: On the changing tilt of the ecliptic, in Mitteilungen der Astronomischen Gesellschaft, Vol. 62, pp. 201-204.
- Astronomical Almanac for 1984, Washington, D.C., 1983. p. 26
- J. Laskar: New precession formulas valid for more than 10000 years, Astronomy and Astrophysics, 157 (1986), 68
How asterisms differ from constellations
Asterisms are distinct patterns that can be seen in the sky and are useful for locating various celestial objects. These patterns are formed by connecting specific stars with imaginary lines. These stars may belong to the same constellation or different constellations. In other words, an asterism can be a part of a constellation.
For instance, one of the most well-known asterisms is the Great Summer Triangle, which is formed by the bright stars Vega, Deneb, and Altair – the alpha stars of the constellations Lyra, Cygnus, and Aquila, respectively. These three prominent stars in the summer sky create an almost isosceles triangle that is easily visible.
Another famous asterism is the Big Bucket, which is a part of the constellation Ursa Major, also known as the Big Dipper. Although the Big Dipper is a larger constellation, the Big Bucket is a distinct and noticeable section that resembles a bucket.
The asterism known as the “W” of the constellation Cassiopeia is widely recognized and often compared to the letter itself. This well-known asterism is just a small part of the entire constellation.
Another famous asterism is Orion’s belt, which consists of three stars arranged in a straight line. Many people are familiar with this distinctive pattern.
In reality, there are numerous asterisms in the celestial sky. These formations create simple shapes that are easy to remember and help in locating various objects or constellations. For instance, the Summer Triangle can be instantly spotted, and its points feature the principal stars of three constellations. Similarly, Polaris can be easily found using the handle of the Big Dipper.
Capella (α of Ascendant)
Capella, which is the third brightest star in the Northern Hemisphere, holds a unique position among the first magnitude stars. Unlike the famous Polaris, which is only of second magnitude, Capella is positioned closest to the North Pole.

Capella is currently recognized as a remarkable 4-star system, consisting of 2 G-class yellow giants and a pair of smaller red dwarf stars.
The first yellow giant, called Aa, has a luminosity 80 times greater and a mass nearly three times larger than our Sun. The second yellow giant, Ab, is 50 times brighter and 2.5 times heavier than the Sun. When combined, the luminosity of these two yellow giants is 130 times that of our Sun.
Locating the star Capella in the celestial sphere
By mentally connecting the two uppermost stars that form the handle of the Big Dipper constellation, you will inevitably encounter the prominent star Capella. Capella is situated within the irregular pentagon shape of the Ascendant constellation.
For instance, if you find yourself at a latitude of 44 degrees north (Pyatigorsk, Russia) or even further north, you will have the opportunity to observe Capella continuously throughout the night. At these latitudes, Capella never dips below the horizon.
Being in the same part of the world, we observe the celestial dome above us. It encompasses approximately 180 degrees of the entire sphere, which represents our planet Earth.
In other words, individuals in a specific part of the world can only see the constellations that are directly overhead. To view the constellations on the opposite side of the sphere, one must relocate to the other part of the world.
Imagine yourself standing on an orange and gazing upwards. The entire space beneath the orange (in the Southern Hemisphere) remains hidden from your view.
As a result, astronomers categorize constellations based on whether they are visible solely in the Southern Hemisphere or accessible to those residing in the Northern Hemisphere.
The constellations found in the Southern Hemisphere include:
- The Great Dog,
- The Wolf,
- The Raven,
- The Dove,
- The Sacristan,
- The Painter,
- The Crane,
- The Hare,
- Goldfish,
- Indian,
- Kiel,
- Capricorn,
- Compass,
- Feeds,
- Flying fish,
- Microscope,
- Fly,
- Pump,
- Naugolnik,
- Octant,
- Peacock,
- Sails,
- Stove,
- Bird of Paradise,
- Chisel,
- Grid,
- Scorpion,
- Table Mountain,
- Sagittarius,
- Telescope,
- Toucan,
- Phoenix,
- Chameleon,
- Centaurus,
- Circulus,
- Clock,
- Cup,
- Shield,
- Southern Hydra,
- Southern Crown,
- Southern Fish,
- Southern Cross,
- Southern Triangle.
The constellations in the Northern hemisphere include:
- Andromeda,
- Gemini,
- Taurus,
- Pisces,
- Leo,
- Ursa Major,
- Ursa Minor,
- Orion,
- Cassiopeia,
- Cygnus,
- Draco,
- Lyra,
- Perseus,
- Bootes,
- Aquarius,
- Aries,
- Cancer,
- Virgo,
- Libra,
- Scorpio,
- Capricornus,
- Aquila,
- Pegasus,
- Corona Borealis,
- Hercules,
- Canis Minor,
- Canis Major,
- Lynx,
- Leo Minor,
- Draco Cephalus,
- Ursa Minor,
- Perseus,
- Cepheus,
- Cygnus,
- Cassiopeia,
- Vega,
- Altair,
- Deneb,
- Vulpecula,
- Sagitta,
- Delphinus,
- Pegasus,
- Andromeda,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
- Scorpio,
- Sagittarius,
- Capricornus,
- Aquarius,
- Pisces,
- Aries,
- Taurus,
- Gemini,
- Cancer,
- Leo,
- Virgo,
- Libra,
The constellations include the Big Dipper, the Volopassus, Veronica’s Hair, Hercules, Hound Dogs, Dolphin, Dragon, Giraffe, Cassiopeia, Swan, Lyra, Foxy, Little Bear, Little Horse, Little Leo, Aries, Pegasus, Perseus, Cancer, Lynx, Northern Crown, Sextant, Arrow, Triangle, Cepheus, and Lizard.
Ecliptic
The ecliptic is a fictional line (the celestial sphere’s great circle) that the Sun follows as it moves among the stars throughout the year.
This term also refers to the visible path that the Sun travels through the twelve constellations of the zodiac and the Serpentine constellation during the year. It coincides with the line that defines the Earth’s and other planets’ orbital plane around the celestial body.
The term “ecliptic” (derived from the Greek word εικλειπτική, meaning “eclipsed” line) is associated with the ancient knowledge that solar and lunar eclipses occur only when the Moon is near the points where its orbit intersects the ecliptic. These points on the celestial sphere are known as lunar nodes, and the period of their movement along the ecliptic, which lasts about 18 years, is called saros or draconic period.
Most of the planets in the Solar System orbit in a plane called the ecliptic. They also move in the same direction as the Sun’s rotation.
However, the Moon’s orbit is tilted relative to the ecliptic, and because of the Earth’s rotation around the barycenter of the Moon-Earth system, as well as perturbations from other planets, the true position of the Sun can deviate slightly from the ecliptic. This deviation is typically only a few seconds of arc. The path of the “average Sun” can be said to follow the ecliptic.
Coordinate points
In the field of astronomy, the ecliptic serves as the fundamental circle for the coordinate scheme.
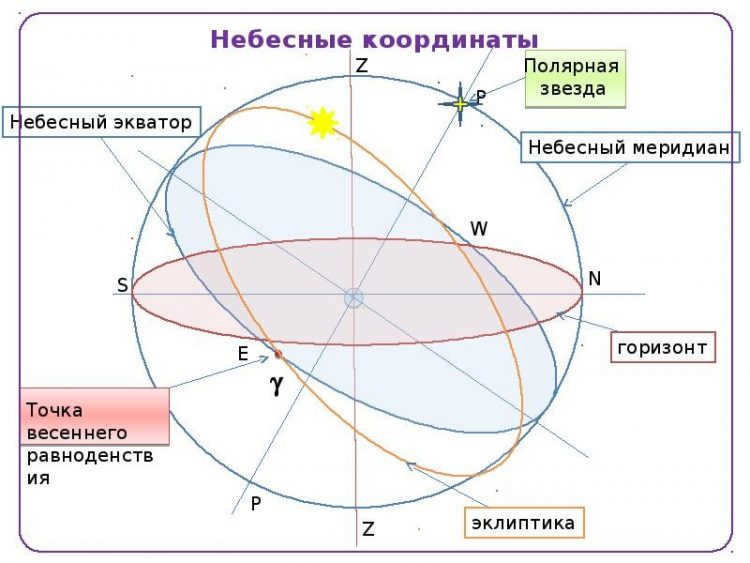
Celestial latitude is determined by measuring the angle from the celestial equator to the north or south pole of the ecliptic. This angle is measured from east to west, with the point where the perpendicular to the ecliptic intersects the celestial sphere serving as the pole of the ecliptic.
The ecliptic divides the celestial equator into two points known as the equinoxes or nodes. The summer and winter solstices are located halfway between these points.
The celestial equator, also known as the equinox line, is a plane that is perpendicular to the Earth’s axis of rotation and passes through its center. On star charts, the celestial equator appears as a line running directly from east to west, following the daily rotation of the stars.
The term “equator” is derived from the phrase “line of equality.” It is given this name because the stars in this region spend an equal amount of time both above and below the horizon. The Sun also experiences this phenomenon twice a year during the equinoxes.
In the ecliptic celestial coordinate system, the ecliptic plane serves as the primary plane.
Not all celestial bodies in the sky remain stationary. They exhibit motion. Although the stars appear to be still when observed, this is not actually the case. In comparison to the moon or the sun, the stars’ movement is barely noticeable. Their speed is incredibly slow, but their distance from Earth is much greater. The movement of a star can only be detected with the aid of a telescope. Even if one were to remain in the same position for several years, the naked eye would not be able to perceive the stars’ motion. The Sun and Bernard’s star, which cannot be seen with the naked eye, have their own rapid motion. Despite only being visible through a telescope, the angular velocity of Bernard’s star is higher than that of other stars.
The movement of heavenly bodies is both daily and yearly. Throughout the daily motion, stars shift their position in relation to the edges of the horizon. The stars exhibit their apparent daily movement along the celestial sphere. This apparent motion is directly linked to the Earth’s rotation on its axis. By observing the Sun, one can distinctly perceive its emergence in the eastern sky during the early morning and its descent beyond the western horizon in the evening. Over the course of a year, it traverses through twelve constellations of the zodiac, spending approximately one month in each.
The apparent movement of the Sun. The path of the Sun
When we look up at the night sky, we can see that as each night passes, more and more stars reach their highest point. This phenomenon occurs because of the Earth’s annual orbit around the Sun, causing the Sun to move among the stars. The Sun’s movement follows the same direction as the Earth’s rotation, from west to east. This path of the Sun’s apparent motion is known as the ecliptic, which forms a large circle on the celestial sphere. The plane of the ecliptic is inclined at an angle of 23°27′ to the plane of the celestial equator and intersects with it at two points called the vernal and autumnal equinoxes. The vernal equinox occurs around March 21st, when the Sun transitions from the southern celestial hemisphere to the northern celestial hemisphere. The autumnal equinox happens around September 23rd, marking the Sun’s transition from the northern hemisphere to the southern hemisphere. Along its journey along the ecliptic, the Sun passes through 12 constellations known as the zodiac belt.
The different parts of the Earth’s orbit around the Sun create varying conditions of illumination and heating on its surface, thanks to the rotation of the planets, including Earth. This phenomenon is responsible for the changing seasons, which can be explained by three factors: the tilt of Earth’s axis in relation to its orbit, the fixed position of the axis in space, and the Earth’s revolution around the Sun. From an Earthly perspective, it appears that the Sun has its own motion across the celestial sphere, but in reality, this motion is caused by the Earth’s rotation.
Fig. II.9: The celestial sphere’s ecliptic plane:
PP¢ – Earth’s poles; l – longitude on the ecliptic; b – latitude on the ecliptic; EE¢ – the ecliptic plane; QQ¢ – the celestial equator; M – the Sun
The motion of the Sun relative to the stars can be observed along a circle on the celestial sphere known as the ecliptic, which is derived from the Greek word for “eclipse”. The ecliptic is inclined at an angle of 23°27¢ to the celestial equator (see Figure II.9). When the Sun reaches the points where the ecliptic intersects with the celestial equator, day and night are of equal length on Earth. These points are known as the vernal equinox (March 21) and the autumnal equinox (September 23). At these points, the Sun’s coordinates, declination (d) and right ascension (a), are both zero. The uppermost point of the ecliptic, also known as the point of the summer solstice (June 22), corresponds to a right ascension of 6 hours and a declination of +23°27¢. Conversely, the lowest point of the ecliptic, reached by the Sun on December 22, is called the point of the winter solstice and has a right ascension of 18 hours and a declination of -23°27¢. The Sun moves along the ecliptic at a rate of approximately 1° per day. The time between two passes of the Sun at the vernal equinox is known as a tropical year, which lasts for 365.2422 days.
Due to the Moon’s gravitational influence, the Sun reaches the vernal equinox 20 minutes and 24 seconds earlier each year compared to when the Earth completes another orbit around it. This phenomenon is known as precession, or the prevarication of the equinoxes. As a result of precession, the Earth’s axis of rotation undergoes a rotation of 50.27 arcseconds per year, tracing out a conical path in space. Interestingly, the Earth’s axis will complete a full revolution around the ecliptic axis in 25,800 years. This period of precession plays a significant role in understanding long-standing climate changes on Earth and the formation of ice ages.
LIST OF RECOMMENDED LITERATURE
1. Orlenok V.V. Physics of the Earth, Planets, and Stars. Kaliningrad, 1991. 175 p.
>Chapter III. Internal Structure of the Earth
The Exciting Company
In an effort to revolutionize transportation on the Red Planet, a groundbreaking initiative was launched in 2016. Elon Musk, the visionary behind SpaceX, created The Exciting Company as a spin-off dedicated to the development of advanced underground infrastructure. This technology focuses on the construction of transportation tunnels, with the primary goal of facilitating the future Hyperloop trains. The inaugural tunnel, situated 4.6 meters below the surface, was excavated within the premises of SpaceX’s offices in Hawthorne. Interestingly, this location allowed for construction without the need for additional permits.
The ultimate objective of The Exciting Company is to address traffic congestion and enable swift point-to-point travel, leading to the transformation of cities.
Achievements: the team has successfully created and constructed their very own innovative Prufrock tunnel boring machine. Interestingly, this machine is compact enough (with a diameter of 3.7 meters) to be transported on board the SpaceX Dragon spacecraft. As part of their groundbreaking research, experimental tunnels have been constructed in both Las Vegas and Los Angeles. Additionally, there are numerous upcoming projects in the cities of Los Angeles, Chicago, and Baltimore, pending approval from the relevant authorities.
The Stars’ Movement: A Trick of the Eye
The apparent motion of the stars across the night sky is nothing more than an optical illusion, created by the Earth’s rotation on its axis. The direction in which they appear to move depends entirely on the perspective of the observer.
When facing north, it seems as though the constellations are circling around a fixed point in the sky known as the north pole, which is near Polaris. This perception is a result of the Earth spinning from west to east, causing the ground beneath us to move to the right. As a consequence, the stars, including the sun, moon, and planets, appear to move in an east-west direction, from right to left. However, if we were to turn and face south, the stars would appear to move in a clockwise direction, from left to right.
Relationship to the Celestial Equator
The celestial equator is a reference plane that is formed by projecting a plane from Earth’s orbit in all directions. This plane, known as the ecliptic, is shown projected outward (gray) on the celestial sphere, along with Earth’s equator and polar axis (green). The ecliptic intersects the celestial sphere in the form of a great circle (black), which is the same circle that the Sun appears to orbit around the Earth. The points where the ecliptic and equator intersect on the celestial sphere are the spring and fall equinoxes (red), which mark the moments when the Sun appears to cross the celestial equator.
Orientation The earth’s axis and equator undergo a continuous rotation around the pole of the ecliptic, a process known as the luni-solar precession, which takes approximately 26,000 years. This rotation is primarily caused by the gravitational influence of the moon and sun on the Earth’s equatorial bulge. Likewise, the ecliptic itself is not stationary. The gravitational interactions with other celestial bodies in the solar system result in a slight movement of the Earth’s orbital plane and, consequently, the ecliptic plane. This phenomenon is referred to as planetary precession. The combined effect of the luni-solar precession and planetary precession is known as General precession, which causes the equinoxes to shift their position by approximately 50 angular seconds (equivalent to about 0.014 degrees) every year.
Once more, this is a simplification. The cyclical movements of the Moon and the apparent cyclical movements of the Sun (actually the Earth in its orbit) lead to short-term cyclical small amplitude oscillations of the Earth’s axis and thus the celestial equator, known as nutation. This introduces a cyclical element to the positions of the equinoxes; the positions of the celestial equator and the (vernal) equinox with fully updated precession and nutation are referred to as the true equator and equinox; the positions without nutation are called the mean equator and equinox.

When reading popular science articles about space and astronomy, you may come across the term “ecliptic,” which can be a bit confusing. This term is commonly used by both scientists and astrologers to describe the position of distant objects in relation to the solar system and the orbits of celestial bodies within it. So, what exactly is the “ecliptic”?
What is the connection to the zodiac?
The ancient astrologers who studied the movements of the celestial bodies made an interesting observation regarding the Sun. They noticed that the Sun appeared to move in relation to the stars. By carefully monitoring its trajectory across the sky, they discovered that every year, the Sun returns to its original position. Furthermore, they observed that the Sun follows the same path, known as the ecliptic, year after year. This path represents the journey of our primary luminary throughout the calendar year.
According to the beliefs of the ancient Greeks, the Sun, personified as Helios, travels through the constellations in his majestic golden chariot pulled by golden horses.


The zodiac is a ring of twelve constellations that the Sun passes through, and these constellations are referred to as zodiacal.
If your astrological sign is Leo, you won’t be able to spot this specific constellation in the night sky during July, the month associated with Leo. However, during this time period, the Sun is positioned in the Leo constellation, meaning you may have a chance to see it if you’re fortunate enough to witness a total solar eclipse.
By observing the night sky in the daytime (not just during a total solar eclipse, but also with a regular telescope), it becomes apparent that the Sun occupies a specific position within one of the zodiac constellations. For instance, in November, it is likely to be situated in Scorpio, whereas in August, it will be found in Leo. The following day, the Sun’s location will have slightly shifted to the left, and this pattern will repeat each day. After a month (on November 22), the Sun will eventually cross the border of Scorpio and enter the realm of Sagittarius.
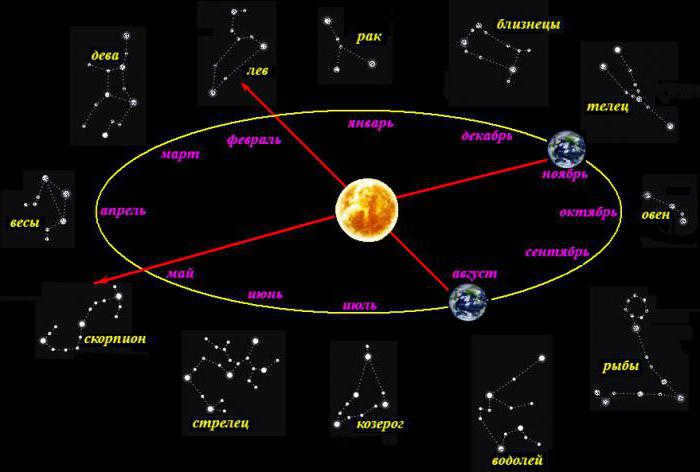

During the month of August, it becomes evident in the diagram that the Sun will be located within the boundaries of the Leo constellation. This pattern continues throughout the year, as each day the position of the Sun is marked on a star chart, resulting in a map with a completed ellipse. Thus, the ecliptic is defined as this specific line.
When to Make Observations
To make observations of their constellations (the zodiac signs under which a person is born), it is best to do so in the month opposite to their date of birth. This is because the ecliptic, which is the path of the Sun, causes the constellation to be high above the horizon at noon for people born in August under the sign of Leo. Therefore, it is difficult to see the constellation during daylight hours when the sunlight is too bright.
However, come February, Leo will be adorning the midnight sky. On a night devoid of moonlight and clouds, it stands out prominently amidst the backdrop of other celestial bodies. Those born under the sign of Scorpio, on the other hand, aren’t as fortunate. The best time to observe this constellation is in the month of May. However, it requires both patience and luck to catch a glimpse of it. It is advisable to venture out into the countryside, away from tall mountains, trees, and buildings. Only then will the observer be able to make out the distinct shape of Scorpius, highlighted by its striking ruby-hued star, Antares (also known as alpha Scorpius). This bright, blood-red star falls into the category of red giants, boasting a diameter comparable to the size of our neighboring planet, Mars’ orbit.
What is the reason for using the term “ecliptic plane”?
Not only does the ecliptic describe the path of the Sun’s yearly movement, but it is also commonly referred to as a plane. When discussing the location of celestial objects and their orbits in space, you will frequently hear the term “ecliptic plane.” Let’s delve into its meaning.
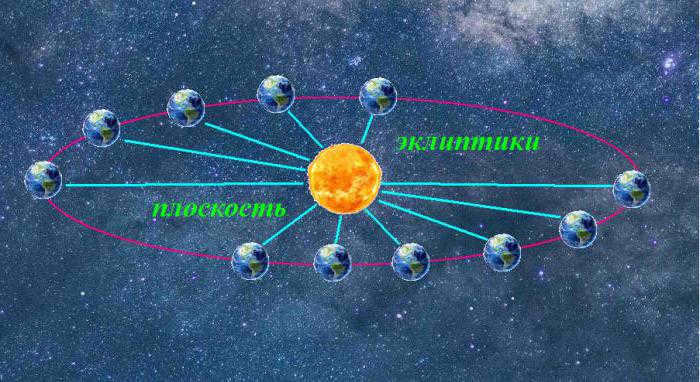
When we consider the orbit of our planet around the Sun and the lines connecting the Earth to the Sun at different moments in time, we find that they all lie in the same plane known as the ecliptic. This plane can be imagined as a disk, with the 12 constellations positioned along its sides. If we draw a perpendicular line from the center of this disk, it will intersect with a point on the celestial sphere in the northern hemisphere. This point is located near the constellations of Ursa Major and Ursa Minor, also known as the Big and Little Dipper.
As we know, the Earth’s axis of rotation is tilted at an angle of 23.44 degrees relative to the ecliptic axis. This tilt is responsible for the changing of seasons on our planet.
And our “neighbors”
Here is a concise overview of the concept of the ecliptic. In the field of astronomy, there is a fascination with the movements of other celestial bodies within our solar system. Through calculations and observations, it has been determined that all the major planets orbit the Sun in a nearly identical plane.
Mercury, being the closest planet to the Sun, deviates from the ecliptic plane by an angle of approximately 7°.
Among the outer planets, Saturn has the largest inclination angle in its orbit (around 2.5°). However, considering its vast distance from the Sun – which is ten times farther than that of Earth – this deviation is quite forgivable for the giant of our solar system.
On the other hand, smaller celestial bodies such as asteroids, dwarf planets, and comets exhibit much greater deviations from the ecliptic plane. For instance, the dwarf planet Erida, which is a twin of Pluto, has an incredibly elongated orbit.